 Alfred Ajibola
- Sat, 22nd February, 2020 @ 9:55 PM
Alfred Ajibola
- Sat, 22nd February, 2020 @ 9:55 PM
Topics in Mathematics
Angles: Type of angles based on degree measurement Angle Pairs: Types of angle pairs Conversion of Number Bases explained with examples Angles: What are Angles? Categories of Angles Lines and Types in Mathematics Number Base System - Table showing the Number Bases with explanations Mathematics Scheme of Work, SS1, 3rd Term Mathematics Scheme of Work, SS1, 2nd Term Mathematics Scheme of Work, SS1, 1st Term Trigonometry - Sine, Cosine and Tangent explained with worked examples Quadratic Equation - Factorization of Quadratic Equation Algebra - Explanation and Worked Examples on AlgebraAcademic Questions in Mathematics

What type of polygon is shown in the above image?
A. Nonagon
B. Decagon
C. Octagon
D. Hexagon
E. Hepagon
F. Pentagon
Which of the following is not part of a circle?
A. Section
B. Sector
C. Segment
D. Semicircle
E. Secant
F. Center

What angle is shown in the above image?
A. Complementary
B. Supplementary
C. Acute
D. Obtuse
E. Reflex
F. Straight
An angle greater than 90o but less than 180o is termed _____.
A. Complementary
B. Supplementary
C. Acute
D. Obtuse
E. Reflex
F. Straight
Factorize 12a2 - 20a + 3
A. (6a - 1)(2a - 3)
B. (6a - 1)(2a + 3)
C. (6a + 1)(2a - 3)
D. (6a + 1)(2a + 3)
E. (-6a + 1)(2a - 3)
F. (-6a + 1)(2a + 3)
Simplify 10ab + 7ab
A. 17ab2
B. 17a2b2
C. 3ab2
D. 3a2b2
E. 17ab
F 3ab
Which of the following is not a type of angle?
A. Right angle
B. Left Angle
C. Acute Angle
D. Complete Angle
E. Straight Angle
F. Reflex angle
Two lines that can never meet each other are best considered as _____ lines.
In order to convert a base to another base; for instance, if we were to convert a base 10 to base 2, we will first need to know the powers of the base to which we are converting to.
Please read on the introduction and explanation on number base system using tables here.
The instances below shows the powers of various numbers:
The powers of 2 are:
And so on.
Any value raised to the power of zero will always equal to 1.
Simply put, the power of 2 can be written as:
Following the same steps as above, the power or 3 will be:
Still following the same steps as above, the powers of 4 are:
You can read on the factorization of quadratic equation here.
Note: During the final step of our base conversion, the value 'one' (1) divided by our base value of interest will always result in the answer 'zero remainder one' (0 remainder 1). This is an important step to note as we shall see in our examples below.
Step 1: We need divide the decimal number by the base we want to convert to.
Note: We must take into account the remainder from our division because it will be used as our answer.
Every other step will be similar to step 1 but we will divide our resultant answer from the subsequent steps.
Step 2: Our previous answer from the above division was 5
Step 3: Our previous answer from the above division was 2
Step 4: Our previous answer from the above division was 1
Once our division equals 0, we are done.
Next; we will have to write the reminder from bottom - top; and that’s our answer.
Answer is 10102
OR
We can also solve this question by using a table; where we will divide the decimal number by the base we want to convert to:
|
Base 2 |
Decimal value |
Remainder |
|
2 |
10 |
- |
|
2 |
5 |
0 |
|
2 |
2 |
1 |
|
2 |
1 |
0 |
|
2 |
0 |
1 |
Counting our remainder from below;
Answer is 10102
Please read on the explanations and worked examples on algebra here.
Note: I will use different expressions for the questions below but they will all mean the same thing. (We will be converting a number from base 10 to another base).
For simplicity, we will use a table to solve this question.
|
Base 2 |
Decimal value |
Remainder |
|
2 |
15 |
- |
|
2 |
7 |
1 |
|
2 |
3 |
1 |
|
2 |
1 |
1 |
|
2 |
0 |
1 |
Counting our remainder from below;
Answer is 11112
You can read on trigonometry explained with worked examples here.
|
Base 4 |
Decimal value = 16 |
Remainder |
|
4 |
16 |
- |
|
4 |
4 |
0 |
|
4 |
1 |
0 |
|
4 |
0 |
1 |
Counting our remainder from below;
Answer is 1004
|
Base 16 |
Decimal value = 16 |
Remainder |
|
16 |
16 |
- |
|
16 |
1 |
0 |
|
16 |
0 |
1 |
Counting our remainder from below;
Answer is 1016
Kindly share this article via the links below:

Please click here to contact Alfred if you require any of the following services:
If you need a standard website at an affordable price.
Online training on the academic subjects: biology, chemistry and basic science.
If you require an advanced smart school management system (web application) for your school.
Click here to read on Len Academy Smart School Software.
Please click here to follow Len Academy on Google News.
Amazing facts in Mathematics
The opposite sides of a dice will always give a sum of seven
31,556,926 seconds makes one year
From 0 through 1000, the only number that has the letter 'a' in it is 'one thousand'
÷
The division symbol '÷' is actually called an obelus
Four is the only number in the English language that is spelt with the same number of letters as the number (4) itself
40 is spelt as forty and not 'fourty'. It is the only number that is spelt with letters arranged in alphabetical order
An odd number is a number that cannot be divisible by 2.
Every odd number has an 'e' in its spelling. They include:
555 in Thailand sounds like 'hahaha'. This is because the number '5' means 'ha' in Thailand
In mathematics, 10! means 10 factorial. Below is its calculation:
10! = 10 x 9 x 8 x 7 x 6 x 5 x 4 x 3 x 2 x 1 = 3628800
Interestingly, 3628800 seconds is the same as 42 days
42 days is the same as 6 weeks
We can therefore say that 10! equals 6 weeks or 3628800 seconds in mathematics
When you multiply 111,111,111 by 111,111,111; the result equals 12,345,678,987,654,321
Notable points in Mathematics
Let's use algebra to explain the simple statement below:
I am a number. When you subtract 3 from me, the result is 9. what number am I ?
Of course we know the answer is 12. You probably guessed it from your mind because it was that simple. The truth is, We could have a much more complicated statement of such; and that is where algebra comes into play.
Now, to apply algebra in the above statement, we use letters or alphabets to represent the unknown number(s). So using algebra, we can write:
Let the number be y
y - 3 = 9
y = 9 + 3
y = 12

The image above shows a right angled triangle. The angle of the above right triangle is denoted as θ. Below are some points to note:
Hypotenuse is always the longest side of the triangle.
Opposite is the side opposite the angle (θ)
Adjacent is the shorter side next to the angle (θ)
Each of Sin θ, cos θ and tan θ are the ratio of sides of the right triangle. They are calculated by using the formula (margic word) sohcahtoa:
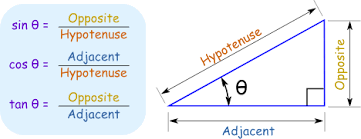
soh
Sin θ = Opposite/Hypotenuse
cah
Cos θ = Adjacent/Hypotenuse
toa
Tan θ = Opposite/Adjacent
Circumference in mathematics means the distance around the edge of a circle (or a another curved surface).
Circumference is to circle or any other curved surface
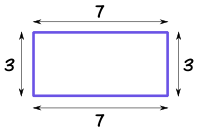
Perimeter means the distance around a square or rectangle or polygon.
Perimeter is to square or rectangle or polygon